Port-based approach of complex distributed-parameter system models for analysis and simulation (PACDAS, STW-TWI.6012)

Elastic film contact problem

Elastic sheet material is modelled with a nonlinear FEM model. The static solution is recovered by treating the problem as a damped dynamical Hamiltonian system. The sheet is fixed at the sides, and a sharp parabolic contact is pressed against it from below. The contact is modelled as a stiff potential. Linear interpolations are used.

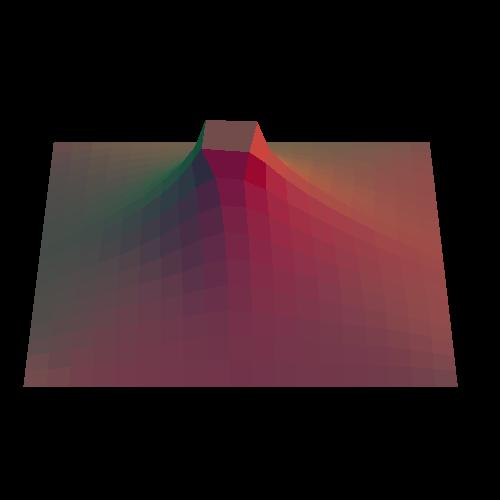
In the center, for large forces, the solution "fails" as the sharp parabolic contact shape (not drawn) presses through a hole in the mesh in the center. It would correspond to tearing a hole in a net. The contacts are only calculated for the mesh nodes (20x20). The second movie shows this effect. Each stationary state takes about a 30 seconds on a 3.4GHz pentiumIV. Due to the hard contact problem the convergence is limited by the small time step (t = 1/K).

An animation of a sequence of stationary solutions for different contact configurations: movie.mpg (150 kb) (generated using ppmtompeg). A similar animation, but with the contact shape added movie2.mpg (205 kb) as a lighter object.

The object of the elastic film is a Hamiltonian. It can be used in many different settings. For example, pressure, gravity, and inertia can be added without problems. Furthermore, boundary conditions are easily changed. For example, the film only attached at the corners in movie3.mpg (290 kb) . The modelling is an attempt to combine constraints, i.e., the contact object, with dynamical features for a consistent simulation of the whole. Furthermore, "failures", such as the contact object pressing through the mesh, are studied to remove finite-size errors, without adding to the numerical complexity.

A three times wider contact shape does not lead to the same tearing problems, showing this is a typical mesh-size problem: movie4.mpg (450 kb) . The convergence is slower, because the film moves only slowly across the contact shape, yielding a slow oscillation. Note, no friction is added to the contact problem: the film moves, in principle, frictionless. The damping is added explictly, as part of the numerical scheme. The kinetic energy serves as convergence test.

In the case of a practical mould (600x800x500mm) the contour levels are given at the left-hand side. The deformation of the elastic film is given on the right-hand side. The film is kept fixed at all the boundaries. The deformations are mainly local; near steep parts the film is stretched. The white lines indicate the stretching. The effects are suprisingly limited.